This question was reportedly poorly attempted by majority of the candidates. According to the
report, majority of the candidates exhibited poor understanding of the topic, judging from their
responses.
Candidates were expected to apply two theorems, to solving this problem. They are:
(1) A straight line drawn from the centre of a circle to a tangent to the circle is perpendicular to
the tangent.
(2) The distance from the centre of a circle to any point on its circumference is constant.
This is illustrated below:
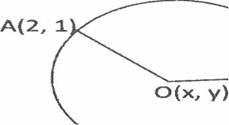
From the diagram,∠OBT = 90° and /0A/ = /OB/. Hence, candidates were expected to show
that since /0A/ = /OB/, then (x - 2)² + (y - 1)² = (x - 4)² + (y + 5)² expanding this equation and
simplifying gave x - 3y = 9. Also,OB and BT are perpendicular and slope of BT = 2.
Therefore, (slope of OB) x (slope of BT) = -1. i.e. y+5 = -!. Simplifying
x-4 2
this equation, we have 2y + x = -6. By solving x - 3y = 9 and 2y + x = -6 simultaneously,we obtain the coordinates of
the centre (x,Y) as (0, -3). Radius of the circle =√(0 - 2)² + (-3 - 1)² =√20.
Thus the equation of the circle = (X-0)² + (y + 3)² = √20. After a little computation, we
obtained X² +y² + 6y-11 = o.



