Question 4
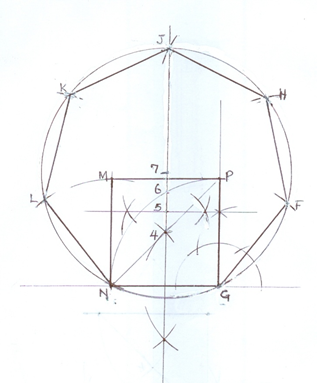
(a) Construct a square and a heptagon on a common base of 40mm.
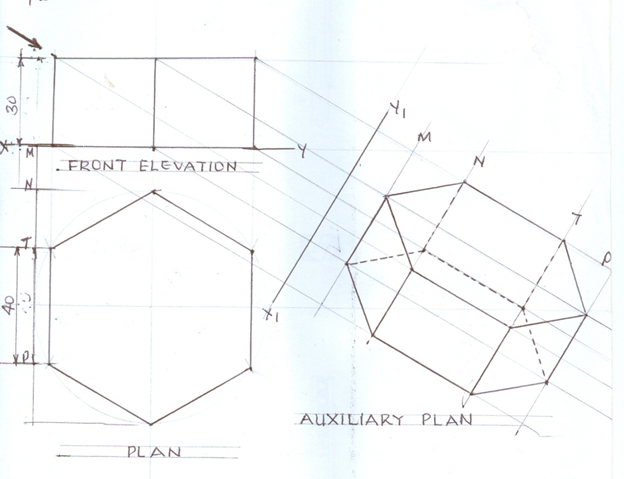
(b) The front front elevation and the plan of a regular hexagonal prism are shown below. Draw the auxiliary plan on plan X1Y1. Show all hidden details.

Observation
This question require candidates to construct a square and heptagon using general method for both on a common base.
Most of the candidates attempted this question and got it right.
(A)CONSTRUCTING THE SQUARE AND HEPTAGON
Candidates were expected to:
(i)draw the given base NG = 40;
(ii)construct the square, MNGP on line NG;
(iii) bisect line NG and drawing its perpendicular bisector;
(iv)draw the diagonal NP or a line at 45o to NG to intersect the bisector at point 4;
(v)draw arc NP with radius NG at G or a line at 60o on NG to intersect the bisector at point 6;
(vi)bisect the distance between points 4 and 6 to locate point 5;
(vii)use the distance between points 4 and 5 (or 5 and 6) to Point 7;
(viii)use radius N7 or G7 at point 7 to describe a circle;
(ix)use side NG as radius to mark off points on the circle and joining the points into a heptagon;
(x)draw neatly.
The solution is shown below
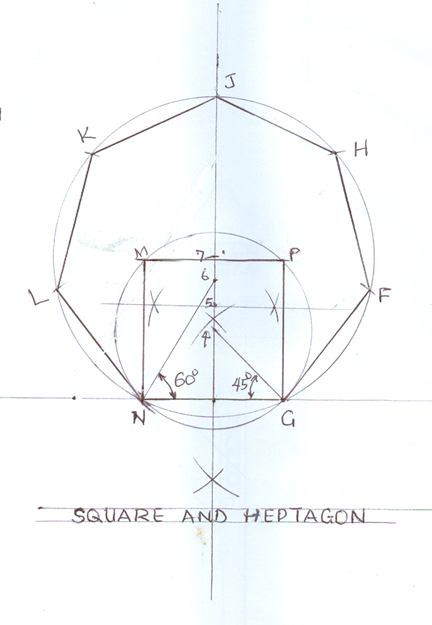
ALTERNATIVE METHOD
(B) This question requires candidates to draw the auxilary plan of a given component.
The three major steps involved are:
- correctly locating the X1Y1 axis
- projecting lines from the front elevation
- transferring ordinate dimension from the plan to draw the auxiliary plan
Most of the candidiates that attempted tis question did not get it right.
AUXILIARY PLAN
Candidates were expected to:
- draw the given plan;
- project from the plan to draw the front elevation;
- draw the given elevation;
- draw the axis X1Y1 at 60o to the horizontal;
- project lines from the front elevation at 90oto the X1Y1axis;
- transfer the corresponding ordinates from the plan to the X1Y1axis;
- locate the points of the auxiliary plan and join the points for the auxiliary plan;
- indicate all hidden details;
- draw neatly
The solution is shown below: