Part (a) of this question was reportedly attempted by very few candidates.    Teachers are encouraged to put in more effort in teaching this topic.  Candidates were expected to apply the ratio theorem in order to obtain the position vector of k as 3  + 2   =   +   = 8.2i + 5.2j
                                       2 + 3              5             
More candidates attempted part (b) and majority of them performed well.  Many of them could draw the correct diagram.  However, some of them were not able to apply the sine and cosine rules correctly to calculate the required distance /AC/ and the bearing of C from A.  The expected diagram was

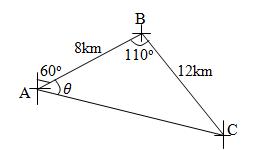
From the diagram, /AC/ could be calculated using the cosine rule,      /AC/2 = 82+122 - 2(8)(12)cos 110° to obtain /AC/ = 16.543km.  The bearing of C from A was determined from the sine rule:

sin = sin110o ,
  12      16.543     

which gave q = 43°. Hence, the bearing of C from A= 60° + 43° = 103o