The Chief Examiner reported that this question was attempted poorly by majority of the       candidates. According to the report, they showed poor understanding of trigonometric       ratios. Candidates were expected to recall that cos 2θ = cos2θ – sin2θ = (1 – sin2θ) – sin2θ = 1       - 2 sin2θ. Hence, sinθ + cos 2θ = 0 becomes sinθ + 1 - 2 sin2θ = 0 which is equivalent to
      2 sin2θ – sinθ - 1 = 0. By substituting y for sinθ, the equation becomes 2y2 – y – 1 = 0. Solving       this quadratic equation correctly gave y = sinθ = 1 or 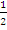. This implied that θ = 90o, 210o or        330o. Hence, the truth set was {90o, 210o, 330o}.