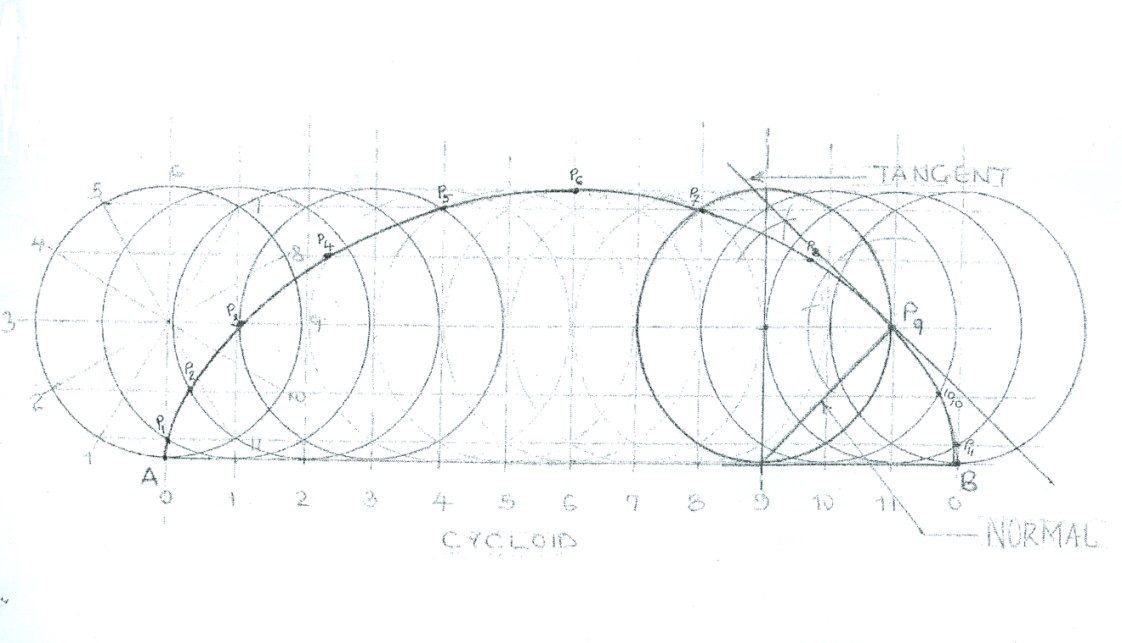
This was a question on drawing the cycloid of a circle, it requires the candidates to locate the different position of the point P on the circle as it rolls along the horizontal line.

 

 Most candidates did not attempt this question, few that attempted it showed weakness in the following areas:

• tracing the different positions of the circle;
• locating the different positions of the point P;
• drawing the curve;
• drawing the tangent;
• constructing the perpendicular at point P.

Candidates were expected to do the following:

A.         DRAWING THE CYCLOID

            (i)         draw the given circle Ø30 and divide it into 12 equal parts;
(ii)        draw line AB equal to the circumference and divide in into 12 equal parts;

• draw vertical lines through the points on line AB;
• draw horizontal lines through the points on the circumference of the circle;
• use the rolling circle to locate various positions of point P;
• join the points into a smooth curve.

B.         DRAWING THE TANGENT

• locate point of tangency at three-quarter turn;
• join P to point 9;
• construct perpendicular at point P (Tangent);
• draw the tangent in outline;

 

The solution is shown below: