The Chief Examiner reported that this question was popular among the candidates and their performance was described as fair. Candidates were expected to represent the given information on a Venn diagram and label the diagram appropriately as shown
From the diagram, x (the number of those who passed all the subjects) = 19 – ( 5 + 9 + 2) = 3, where 19 is the number who passed in Accounts. Those who passed in Mathematics and Economics = 18 – (6 +5 + 3) = 4 while those who passed in Economics only = 16 – 4 – 3 – 2 = 7. Therefore, number who passed in at least one subject =
n(M) + n(E) + 9 – x – 4 = 18 + 16 + 9 – 3 – 4 = 36. This implied that number who failed all the three subjects = 40 – 36 = 4. Number who failed in at least one of Economics and Mathematics = 40 – n(M
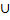
E) = 40 – 27 = 13. Percentage who failed in at least one of Economics and Mathematics =
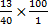
= 32½ %. Number who failed in Accounts = 40 – n(A) = 40 – 19 = 21. Probability of selecting one who failed in Accounts =
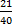
or 0.525.
It was observed that majority of the candidates did not draw the Venn diagram correctly. This affected their correct interpretation and hence the solution of the question.



