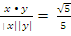The Chief Examiner reported that this question was not attempted by majority of the candidates and majority of those who attempted it performed poorly. In part (a), candidates were expected to recall that if the cosine of the angle between   x = 3i – j and y = 2i +kjwas , then using the dot product, . x • y = (3i –j) • (2i + kj)=  6 – k, |x| =  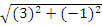=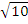, êyê= 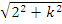= 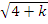2. .

Therefore,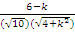=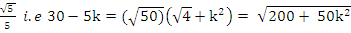. By squaring both sides of the equality sign, we obtained(30 – 5k)2 = 200 + 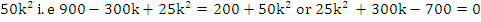. Solving this quadratic equation gave k = 2 and – 14.

In part (b), candidates were expected to prove that if ABCD was a parallelogram, then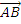// 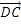and 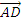//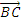.  Now,  =  -  =

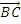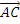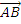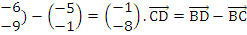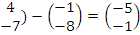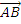(=  ( = . This implied that 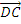//. Similarly,  =  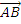+  = = 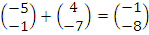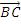. This implied that 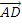//. Therefore, ABCD was a parallelogram.