Question 9
(a) (i) Define dew point.
(ii) Explain why dew forms more quickly on the metal parts than on the rubber parts f a bicycle placed in the open overnight.
(b) (i) Explain the statement, the specific heat capacity of copper is 400 J kg-1 K-1.
(ii) Two metals P and Q are supplied with the same quantity of heat. If the ratio of the specific heat capacity of P to Q is 3:1 and their masses are in the ratio 1:2 respectively, calculate the ratio of the temperature rise of P to Q.
(c) (i) Define coefficient of thermal conductivity of a material.
(ii)
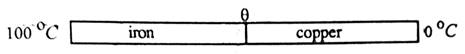
Observation
Part (a): (i) The question was not popular among candidates. Most candidates wrongly presented “dew” as a result of cool. Performance was below average.
(ii) This part was poorly attempted because candidates fail to provide the correct explanation.
Part (b): (i) Performance was below average.
(ii) Very few candidates got the calculation right.
Part (c): Performance was below average because majority of the candidates got the wrong responses.
The expected responses:
(a) (i) Dew Point
The temperature at which the water vapour /moisture present in the atmosphere/air is just sufficient to saturate it
(ii) Explanation of why dew forms more quickly on metal parts than rubber parts
Metal is a better conductor of heat than rubber. At night, metal loses heat faster than rubber and becomes colder. Warm air gains latent heat of vaporization from the metal faster than rubber. Dews are thus formed on the metal parts
(b) (i) Explanation of the Statement
400 J of heat/energy is required to change/raise the temperature of 1kg of copper by 1K.
(ii) Heat = mcDѲ
Hence mpcpDѲp = mQcQDѲQ
Given that cp:cQ= 3:1 and mp:mQ= 1:2
Hence ![]()
Therefore, ![]() :
: ![]() = 2:3
= 2:3
OR
Heat = mcDѲ
mpcpDѲp = mQcQDѲQ
1 x 3 x DѲp= 2 x 1 x DѲQ
![]()
Therefore, ![]() :
: ![]() = 2:3
= 2:3
(c) (i) Definition of Coefficient of Thermal Conductivity
The time rate of flow of heat per unit crosssectionalarea per unit temperature gradient
(ii) (I) ![]()
![]()
![]()
(II) ![]()
= 2.02 Js-1
