Question 1
(1) (a) Draw an ellipse with major axis 120 and the minor axis 70 using the concenric circles method.
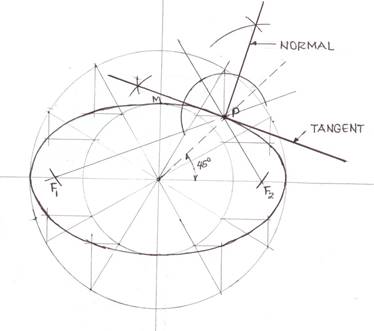
(b) Construct a normal and a tangent at a point P on the ellipse 45o from the centre of the ellipse and at the right side above the horizontal axis.
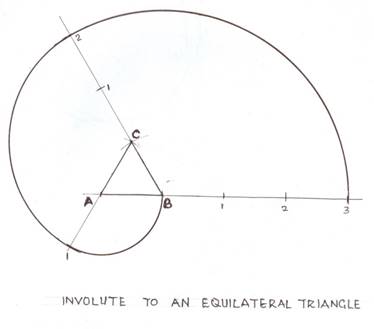
(c) Construct an involute for one complete revolution on an equilateral triangle of side 55.
Question 1A
The candidates were given the major and minor axes and were required to draw an ellipse using the concentric circles method. The solution to this question requires that the candidates draw concentric circles using the major and minor axes as diameters, and then divide the circles into 8/12 equal parts. To locate the points for drawing the ellipse vertical and horizontal lines are drawn from the big and small circles respectively.
Question 1B
This question requires that a point of tangency P is located on the ellipse, and on the point a normal and a tangent is constructed. To solve this, one must locate the local points which are done by using half the major axis as radius and describing arcs on the major axis with the minor axis as the centre.
Question 1C
This is a question that has to do with drawing an involute to an equilateral triangle.
Observation
Question 1A
Many candidates attempted this question and their performance was okay.
Strength: Candidates were able to draw:
- the major and minor axes;
- the major and minor axes at right angle;
- constructing circles using major and minor axes as diameters;
- divide circles into 8/12 equal parts.
Weakness: Some of the candidates were unable to:
- draw vertical lines from divisional points on big circle;
- draw vertical lines from divisional points on small circle;
- locate points for the curve and joining the points to draw the ellipse.
Many candidates attempted this question and only a very few got it right. Many of the candidates failed to get the focal points.
Strength: Most of the candidates were able to do the following:
- locate the point of tangency P at 45o from the centre of the ellipse;
- locate the foci F1 and F2 using half the major axis as radius with M as the centre;
- join F1 and F2 to point P and extending the lines.
Weakness: It was reported that some of the candidates were unable to:
- bisect the external angle at P for the tangent;
- bisect the internal angle at P for the normal.
Question 1B
Candidates were expected to do the following:
(A) CONSTRUCTING THE ELLIPSE
- draw the major and minor axes at right angle;
- construct circles using major and minor axes as diameters ;
- divide circles into 8/12 equal parts;
iv. draw vertical lines from divisional points on big circle;
v. draw horizontal lines from divisional points on small circle;
vi. locate points for the curve and join the points to draw the ellipse.
(B) CONSTRUCTING THE NORMAL AND TANGENT
- locate the point of tangency P at 450 from the centre of the ellipse;
- locate the foci, F1 and F2,using half the major axis as radius with
M as the centre;
- join F1 and F2 to point P and extend the lines;
- bisect the external angle at P for the tangent;
- bisect the internal angle at P for the normal;
- draw neatly.
The solution is shown below:
Question 1C
Many candidates attempted this question but only a few got it right.
Strength: Candidates showed commendable strength in the following areas:
- drawing the given equilateral triangle ABC;
- drawing an arc radius AB with centre A to intercept CA produced in 1.
Weakness: Candidates weakness were shown in the following areas:
- drawing an arc radius C1 centre C to intercept CB produced in 2;
- drawing an arc radius B2 centre B to intercept AB produced in 3;
- neat and tidy line work.
Candidates were expected to do the following:
(C) INVOLUTE TO A TRIANGLE
i. draw the given equilateral triangle ABC
- draw an arc radius AB with centre A to intercept CA produced in 1
- draw an arc radius C1 with centre C to intercept CB produced in 2
- draw an arc radius B2 with centre B to intercept AB produced in 3