|
Question 12
|
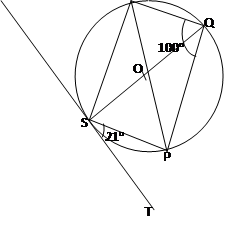
In the diagram, 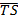 is a tangent to the circle at S. If O is the centre of the circle, ∠TSP = 21o and RQP = 100o, find, with reasons: is a tangent to the circle at S. If O is the centre of the circle, ∠TSP = 21o and RQP = 100o, find, with reasons:
- ∠SPR;
- ∠QSR.
- Given the relation T =
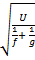 , ,
- Make g the subject of the relation;
- Find g when T = 3, f = 4 and U = 5.
|
|
|
|
|
|



